
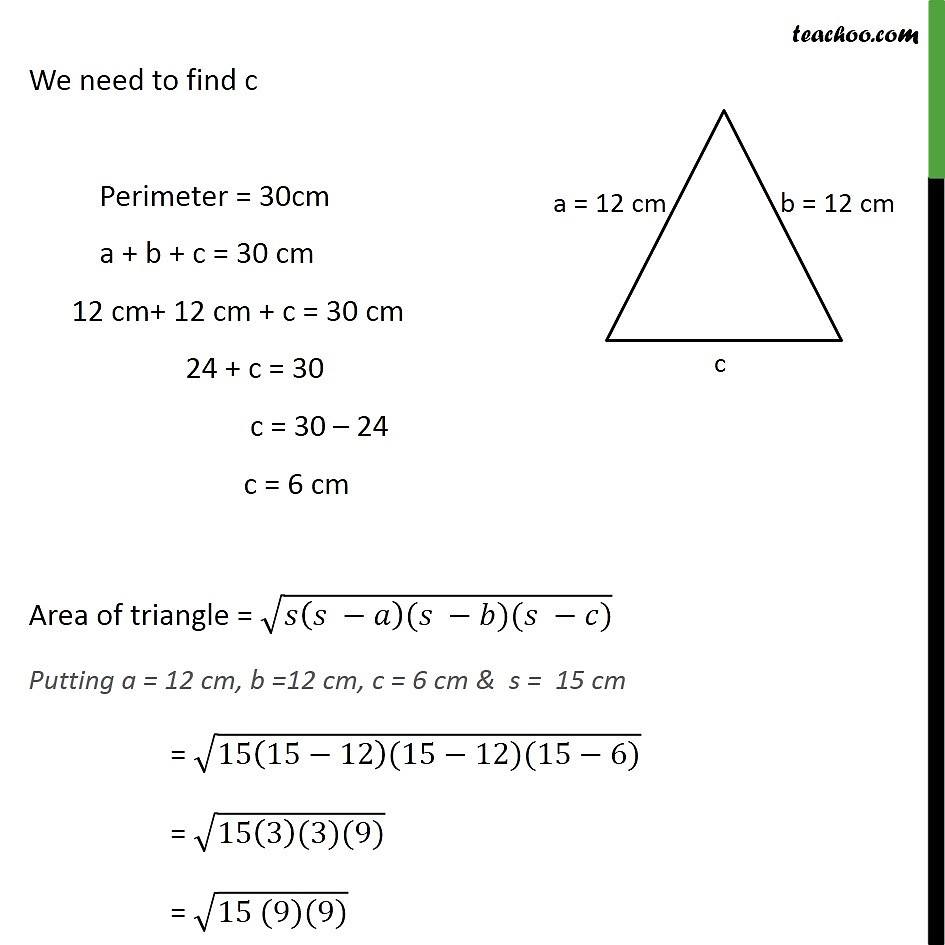
Heron’s Formula for an Isosceles Triangle As long as the three side lengths are known, Heron’s formula works for all triangles. The advantage of Heron’s formula is that no other lengths or angles of the triangle need to be known. Heron’s formula allows us to calculate the area of a triangle as long as all 3 of its sides are known. The formula is named after Heron of Alexandria (10 – 70 AD) who discovered it. It can be used to calculate the area of any triangle as long as all three side lengths are known. Heron’s formula is Area = √( s(s-a)(s-b)(s-c) ), where a, b and c are the three side lengths of a triangle and s = (a + b + c) ÷ 2. This becomes Area = √(10 × 2 × 7 × 1), which simplifies to Area = √140.įinally, the square root of 140 is calculated using a calculator. We find the semi-perimeter by adding up the side lengths and dividing by 2.Ĩ + 3 + 9 = 20 and 20 ÷ 2 = 10. The semi-perimeter is simply half of the perimeter. The first step is to work out the semi-perimeter, s. It does not matter which sides are a, b or c. Substitute the values of s, a, b and c into the formula of Area = √( s(s-a)(s-b)(s-c) ).įor example, find the area of a triangle with side lengths of 8 m, 3 m and 9 m.The steps to find the area of a triangle with 3 sides (a, b and c) are: Simply find the values of s, a, b and c and substitute these into the formula for the area. Heron’s formula is Area = √( s(s-a)(s-b)(s-c) ), where a, b and c are the 3 side lengths of the triangle and s = ( a + b + c) ÷ 2. To calculate the area of a triangle with 3 known sides, use Heron’s Formula. See more information about triangles or more details on solving triangles.How to Calculate the Area of a Triangle with 3 Known Sides Look also at our friend's collection of math problems and questions: Calculate the sides of the triangle.ĪBC isosceles rights triangle the length of each leg is 1 unit what is the length of the hypotenuse AB in the exact form The base is 2 cm longer than the shoulder. The perimeter of the isosceles triangle is 32 cm. Calculate the embankment height.Ĭompute the base of an isosceles triangle, with the arm a=20 cm and a height above the base h=10 cm. The profile of the railway embankment has the shape of an isosceles trapezoid, where a = 16.4 m, c = 10.6 m, and b = d = 5.2 m. What are the angles of an isosceles triangle ABC if its base is long a=5 m and has an arm b=4 m? How many isosceles triangles form in a square when we mark all diagonals?
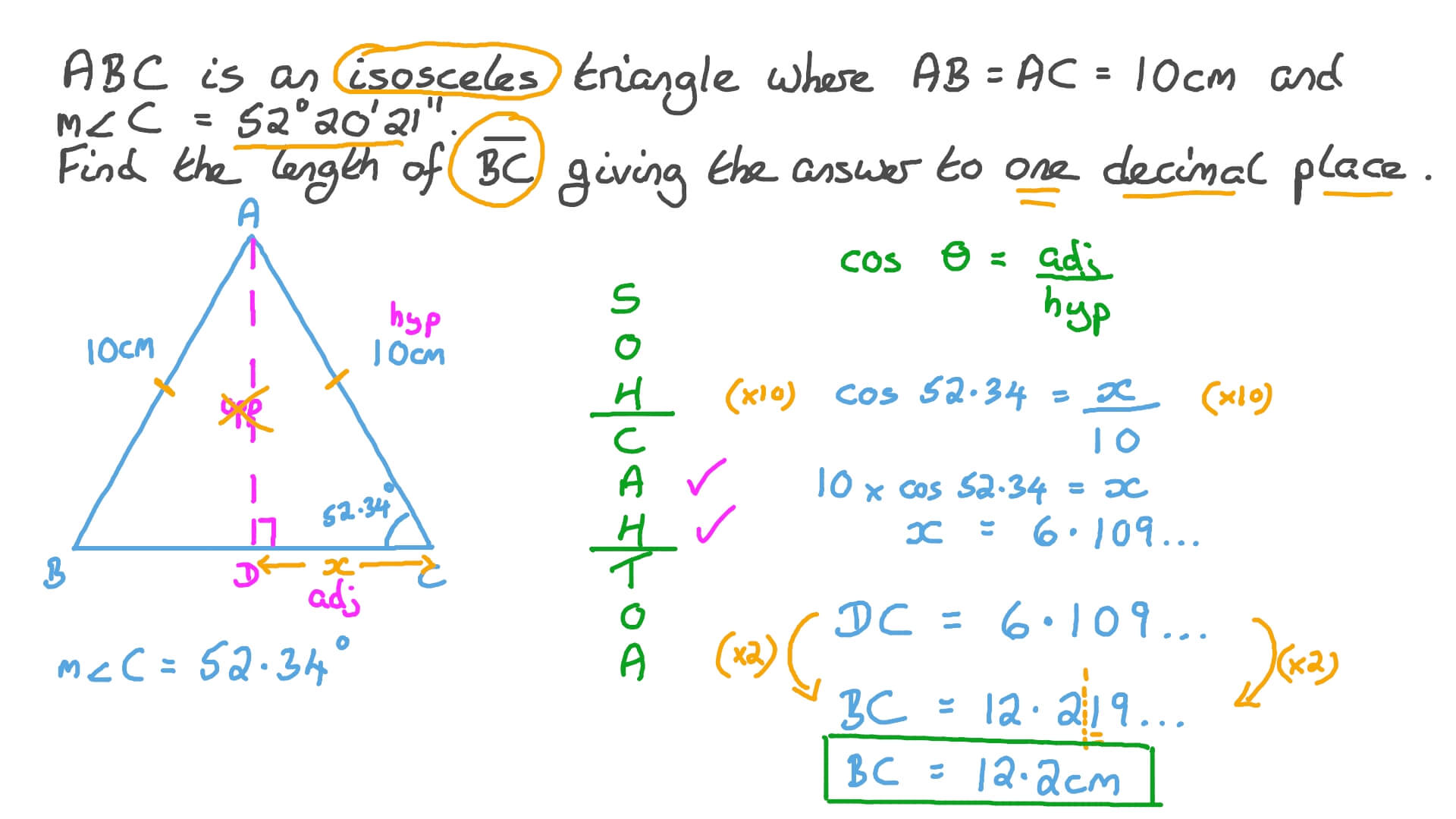
The given is an isosceles triangle with a base of 24dm and an arm of 15dm. How long is a third side?įind the length (circumference) of an isosceles trapezoid in which the length of the bases a,c, and the height h is given: a = 8 cm c = 2 cm h = 4 cm.Īn isosceles triangle with a base of 8 cm. Calculate the radius of the inscribed (r) and described (R) circle.Ĭonstruct an isosceles triangle if a given circle circumscribed with a radius r = 2.6 cm is given.Ĭalculate the area of an isosceles triangle, the base measuring 16 cm and the arms 10 cm.Īn isosceles triangle has two sides of length 7 km and 39 km. In an isosceles triangle ABC is |AC| = |BC| = 13 and |AB| = 10. ( T=12 p=16).Įxamples of calculating isosceles triangles:Īn isosceles triangle in word problems in mathematics:Ĭalculate the perimeter of the isosceles triangle with arm length 73 cm and base length of 48 cm.Īn isosceles triangular frame has a measure of 72 meters on its legs and 18 meters on its base. You can also use the given sides and angles to find the area of the triangle using Heron's formula or using trigonometric functions like Sin or Cos. Once you find the sine of angle A, you can use the inverse sine function (arcsin) to find the measure of angle A in radians or degree. By solving this equation you can find the value of cos(C) and then use the inverse cosine function (arccos) to find the measure of angle C in radians or degree.Īdditionally, you can use the Law of Sines to find the measure of the angles, the formula is: Where c is the length of the non-congruent side, a is the length of the congruent sides, and C is the measure of the angle opposite side c. If you know the lengths of two congruent sides (a,a) and the length of the non-congruent side (c) of an isosceles triangle, you can use the Law of Cosines to find the measure of the angles. To calculate the properties of an isosceles triangle when given certain information, you can use the Pythagorean theorem, the Law of Cosines, or the Law of Sines. An isosceles triangle is a triangle where two sides have the same length. This calculator calculates any isosceles triangle specified by two of its properties.


 0 kommentar(er)
0 kommentar(er)
